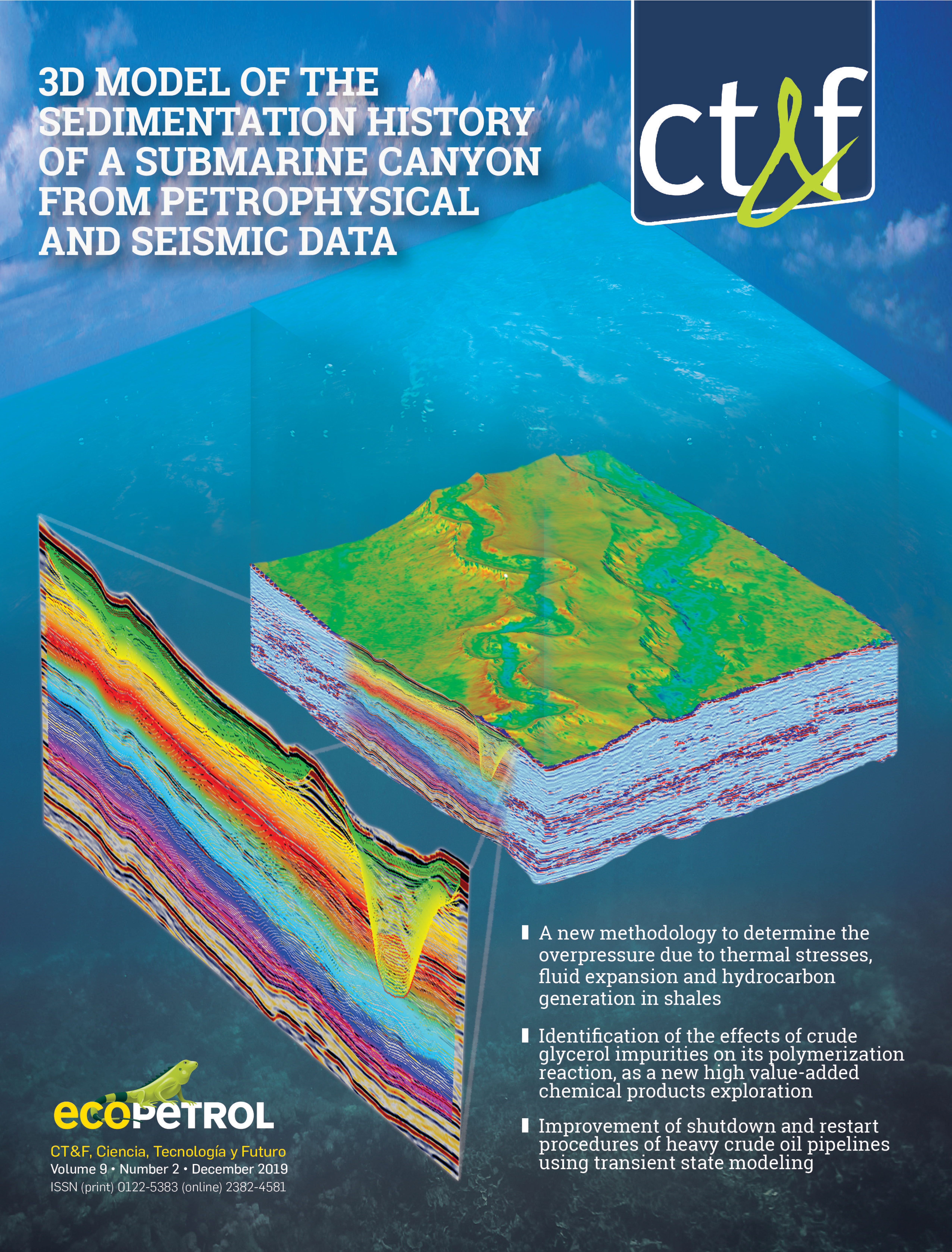
Optimal shape parameter for meshless solution of the 2D Helmholtz equation
Abstract
The solution of the Helmholtz equation is a fundamental step in frequency domain seismic imaging. This paper deals with a numerical study of solutions for 2D Helmholtz equation using a Gaussian radial basis function-generated finite difference scheme (RBFFD). We analyze the behavior of the local truncation error in approximating partial derivatives of the 2D Helmholtz equation solutions when the shape parameter of RBF varies. For discretization, we performed, by means of a classical numerical dispersion analysis with plane waves, a minimization of the error function to obtain local and adaptive near optimal shape parameters according to the local wavelength of the required solution. In particular, the method is applied to obtain a simple and accurate solver by using stencils which seven nodes on hexagonal regular grids, wich mitigate pollution-effects. We validated numerically that the stability and isotropy are enhanced with respect to Cartesian grids. Our method is tested with standard case studies and velocity models, showing similar or better accuracy than finite difference and finite element methods. This is an efficient way for interacting with inverse and imaging problems such as Full Wave Inversion.
References
[2] Ivo M. Babuska and Stefan A. Sauter. Is the pollution effect of the fem avoidable for the Helmholtz equation considering high wave numbers SIAM J. Numer. Anal.,34(6):2392-2423, December 1997.
[3] Zhongying Chen, Dongsheng Cheng, Wei Feng, and TINGTING Wu. An optimal 9-point finite difference scheme for the Helmholtz equation with PML. Int. J.
Numer. Anal. Model, 10:389-410, 2013.
[4] Zhongying Chen, Tingting Wu, and Hongqi Yang. An optimal 25-point finite difference scheme for the helmholtz equation with PML. Journal of Computational
and Applied Mathematics, 236(6):1240-1258, 2011.
[5] Björn Engquist and Andrew Majda. Absorbing boundary conditions for numerical simulation of waves. Proceedings of the National Academy of Sciences, 74(5):1765-1766, 1977.
[6] Gregory E. Fasshauer. Meshfree Approximation Methods with Matlab. World Scientific, 2007.
[7] J.C. Fabero, A. Bautista, and L. Casasus. An explicit finite differences scheme over hexagonal tessellation. Applied Mathematics Letters, 14(5):593-598, 2001.
[8] Bengt Fornberg and Natasha Flyer. A primer on radial basis functions with applications to the geosciences, volume 87. SIAM, 2015.
[9] Daniel T. Fernandes and Abimael F. D. Loula. Quasi-optimal finite difference method for Helmholtz problem on unstructured grids. International Journal for
Numerical Methods in Engineering, 82(10):1244-1281,2010.
[10] Bengt Fornberg, Elisabeth Larsson, and Natasha Flyer. Stable computations with Gaussian radial basis functions. SIAM Journal on Scientific Computing, 33(2):869-892, 2011.
[11] Bengt Fornberg, Erik Lehto, and Collin Powell. Stable calculation of gaussian based RBF-FD stencils. Computers & Mathematics with Applications, 65(4):627-637, 2013.
[12] Gregory E. Fasshauer and Michael J. McCourt. Stable evaluation of Gaussian radial basis function interpolants. SIAM Journal on Scientific Computing, 34(2):A737-A762, 2012.
[13] Jun Fang, Jianliang Qian, Leonardo Zepeda-Nu~nez, and Hongkai Zhao. Learning dominant wave directions for plane wave methods for high-frequency Helmholtz equations. Research in the Mathematical Sciences, 4(1):9, May 2017.
[14] Taeyoung Ha and Imbunm Kim. Analysis of one dimensional Helmholtz equation with PML boundary. Journal of Computational and Applied Mathematics, 206(1):586-598, 2007.
[15] Y.C. Hon and R. Schaback. On unsymmetric collocation by radial basis functions. Applied Mathematics and Computation, 119(2):177- 186, 2001.
[16] Frank D Hastings, John B Schneider, and Shira L Broschat. Application of the perfectly matched layer (PML) absorbing boundary condition to elastic wave propagation. The Journal of the Acoustical Society of America, 100(5):3061-3069, 1996.
[17] Frank Ihlenburg and Ivo Babuska. Dispersion analysis and error estimation of Galerkin finite element methods for the Helmholtz equation. International journal for numerical methods in engineering, 38(22):3745-3774, 1995.
[18] Lise-Marie Imbert-Gerard. Interpolation properties of generalized plane waves. Numerische Mathematik, 131(4):683-711, Dec 2015.
[19] Edward J Kansa. Multiquadric a scattered data approximation scheme with applications to computational fluid-dynamics|ii solutions to parabolic, hyperbolic and elliptic partial differential equations. Computers & mathematics with applications, 19(8-9):147-161, 1990.
[20] Seungil Kim and Joseph E. Pasciak. Analysis of a cartesian PML approximation to acoustic scattering problems in R2. Journal of Mathematical Analysis and
Applications, 370(1):168-186, 2010.
[21] Seungil Kim and Joseph E. Pasciak. Analysis of the spectrum of a cartesian perfectly matched layer (PML) approximation to acoustic scattering problems. Journal of Mathematical Analysis and Applications, 361(2):420-430, 2010.
[22] Elisabeth Larsson, Erik Lehto, Alfa Heryudono, and Bengt Fornberg. Stable computation of differentiation matrices and scattered node stencils based on gaussian radial basis functions. SIAM Journal on Scientific Computing, 35(4):A2096-A2119, 2013.
[23] Leevan Ling, Roland Opfer, and Robert Schaback. Results on meshless collocation techniques. Engineering Analysis with Boundary Elements, 30(4):247-253, 2006.
[24] Leevan Ling and Robert Schaback. Stable and convergent unsymmetric meshless collocation methods. SIAM Journal on Numerical Analysis, 46(3):1097-1115,
2008.
[25] Pankaj Mishra, Sankar Nath, Gregory Fasshauer, and Mrinal Sen. Frequency- domain meshless solver for acoustic wave equation using a stable radial basis- finite difference (RBF-FD) algorithm with hybrid kernels, 4022-4027, 2017.
[26] Pankaj K. Mishra, Sankar K. Nath, Gregor Kosec, and Mrinal K. Sen. An improved radial basis-pseudospectral method with hybrid gaussian-cubic kernels. Engineering Analysis with Boundary Elements, 80(Supplement C):162-171, 2017.
[27] J. W. Nehrbass, J. O. Jevtic, and R. Lee. Reducing the phase error for finite-difference methods without increasing the order. IEEE Transactions on Antennas and
Propagation, 46(8):1194-1201, Aug 1998.
[28] H. Power and V. Barraco. A comparison analysis between unsymmetric and symmetric radial basis function collocation methods for the numerical solution of partial differential equations. Computers & Mathematics with Applications, 43(3):551-583, 2002.
[29] Per-Olof Persson and Gilbert Strang. A simple mesh generator in Matlab. SIAM Review, 46(2):329-345, 2004.
[30] Robert Schaback. Multivariate interpolation and approximation by translates of a basis function. Series In Approximations and Decompositions, 6:491-514, 1995.
[31] J. Strikwerda. Finite Difference Schemes and Partial Differential Equations, Second Edition. Society for Industrial and Applied Mathematics, 2004.
[32] A. I. Tolstykh and D. A. Shirobokov. On using radial basis functions in a "finite-difference mode" with applications to elasticity problems. Computational Mechanics, 33(1):68-79, Dec 2003.
[33] Yi Tao and Mrinal K Sen. Frequency-domain Full Waveform Inversion with plane-wave data. Geophysics, 78(1):R13-R23, 2012.
[34] Holger Wendland. Scattered data approximation, volume 17. Cambridge university press, 2004.
[35] Holger Wendland. On the stability of meshless symmetric collocation for boundary value problems. BIT Numerical Mathematics, 47(2):455-468, Jun 2007.
[36] Grady B Wright and Bengt Fornberg. Scattered node compact finite difference-type formulas generated from radial basis functions. Journal of Computational Physics, 212(1):99-123, 2006.
[37] Man-Wah Wong. An Introduction to Pseudo-Differential Operators. World Scientific Publishing Company, 2 edition, August 1999.
[38] Churl-Hyun Jo, Changsoo Shin, and Jung Hee Suh. An optimal 9-point, finite-difference, frequency-space, 2D scalar wave extrapolator. Geophysics, 61(2):529-537,1996.
Downloads

| Article metrics | |
|---|---|
| Abstract views | |
| Galley vies | |
| PDF Views | |
| HTML views | |
| Other views | |